Skip to main content

Measurement of low and High Resistances(electrical measurement part 02)



In this article, you will find the study notes n Measurement of Low, Medium & High Resistances which will cover the topics such as Classification of Resistance, to Measure Low Resistances are Kelvin Bridge Method, Kelvin-Double Bridge Method,  to Measure Medium Resistances such as Ammeter-Volt Meter Method, Ohmmeter Method, Wheat Stone Bridge Method, to measure high resistances Direct Deflection method, Loss of Charge method & Megha ohm bridge method.

 Classification of Resistance

  • Low Resistance is the range of '0.1โ„ฆ to 1โ„ฆ'.
  • Medium Resistance is the range of 1โ„ฆ to few Megha ohm.
  • High resistance is .1Mโ„ฆ to the higher range.

Methods For Measurement of Low Resistances

  • Kelvin Bridge Method
  • Kelvin Double Bridge
  • Ammeter – voltmeter method

Methods For Measurement of Medium Resistances

  • Ammeter–Voltmeter method
  • Wheat stone bridge method
  • Ohmmeter method

Methods For Measurement of High Resistances

  • Direct Deflection methods
  • Loss of Charge methods
  • Megha ohm bridge methods

Measurement of Low Resistances

When the resistance to be measured is of the order of magnitude of bridge contact and lead resistance, a modified form of Wheatstone’s bridge, the Kelvins Bridge theory is employed.

Kelvin Bridge Method 

Kelvins Bridge theory is a modification of Wheatstone’s bridge and is used to measure values of resistance below 1 ฮฉ. In low resistance measurement, the resistance of the leads connecting the unknown resistance to the terminal of the bridge circuit may affect the measurement.

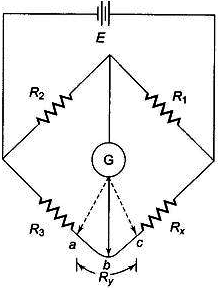
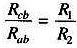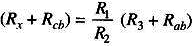
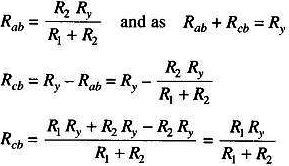
and the usual balance equations for the bridge give the relationship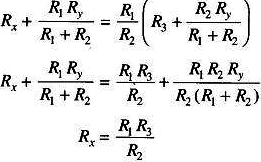Kelvin Double Bridge Method: 

  • Kelvin’s Double bridge is a modification of Kelvins Bridge theory’s bridge and always used in the measurement of low resistance.
  • It uses two sets of ratio arms and the four terminal resistances for the low resistance consider the ckt.
  • The first set of ratio P and Q.
  • The second set of ratio arms are p and q is used to connected to the galvanometer to a pt d.
  • At an Approx. potential between points m and n to eliminate the effects of connecting lead of resistance r between the known std. resistance ‘s’ and unknown resistance R.
  • The ratio P/Q is made equal to p/q. under balanced condition there is no current flowing through galvanometer which means voltage drop between a and b, Eab equal to the
    voltage drop between a and c, Eamd.

I1P=I2 R
I1=I3=E/P+Q 
I2=I4=E/R+S 
E=emf of the battery

If three of the resistances are known then fourth may be determined by formula…

R=S*P/Q

Where R is the unknown resistance, S is called the standard arm resistor and P and Q are called the ratio arms.

Methods For Measurement of Medium Resistances

  • Ammeter–Voltmeter method
  • Wheat stone bridge method
  • Ohmmeter method

Ammeter-Volt Meter Methods

  • We measured the current and the voltage across a variable resistance using the voltmeter-ammeter method, in this method we used two galvanometers with specified range discussed, later on, three-decade boxed the first one is used as the variable resistance Rx, the second and the third are the shunt and series resistances for the ammeter and the voltmeter. 
  • We must know how to convert the galvanometer into a voltmeter or an ammeter. To convert a PMMC into a voltmeter we connect a series resistance with it, the value of this resistance changes according to the value of Rx.

There are two possible connections for the measurement of Medium Resistance using Ammeter Voltmeter Method as shown in the figure below: In both the cases, the reading of Voltmeter and Ammeter is taken.

If the Voltmeter reading is V and Ammeter reading is I then the measured Resistance will be

Rm = V/I

  • This measured Resistance Rm will be the true value of the Resistance if and only if the Resistance of Ammeter is zero and that of Voltmeter is infinite.
  • But actually, this is not possible to achieve zero resistance Ammeter and infinite Resistance Voltmeter. Therefore the measured value of resistance Rm will deviate from the true value R (Say).

Case 1:

The voltmeter is measuring the Voltage drop across the Ammeter as well as the resistor.

It is clear from the figure (1) that Voltmeter is measuring the Voltage drop across the Ammeter as well as the resistor.

So V = Va + Vr

Let current measured by Ammeter = I

Therefore, measured Resistance Rm = V/I

So, Rm = (Va+Vr) / I =(IRa+IR) / I = Ra+R

Therefore, the measured Resistance is the sum of Resistance of Ammeter and true Resistance. Therefore measured value will only represent true value if Ammeter Resistance Ra is Zero.

True value of Resistance R = Rm–Ra= Rm(1-Ra/Rm)

Relative Error = (Rm-R)/R = Ra/R

Note: Relative Error will be less if the true value of Resistance to be measured is high as compared to the internal Resistance of Ammeter. 

Case 2:

The voltmeter is connected in which Voltmeter is connected toward Resistance R whose value is to be measured, Here Ammeter will read the current flowing through the Voltmeter and Resistance R.

 Therefore current measured by Ammeter Ia = Iv+Ir

 So, Ia = Iv+Ir

Measured Resistance

Rm = V/Ia = V/(V/Rv+V/R) = RvR/(R+Rv) = R/(1+R/Rv) 

Therefore, true value of Resistance R = RmRv/(Rv-Rm)

                                                           = Rm(1-Rm/Rv)

Therefore, the true value of Resistance will only be equal to measured value if the value of Voltmeter Resistance Rv is infinite.

If we assume that the value of Voltmeter Resistance Rv is large as compared to the Resistance to be measured R, then Rv>>>Rm

So, True value R = Rm(1+Rm/Rv)

Thus from the above equation, it is clear that the measured value of Resistance is smaller than the true value.

Relative Error = (Rm-R)/R

                = -R/Rv

Therefore, it is clear from the expression of Relative Error that, error in measurement will be low if the value of Resistance under measurement is very less as compared to the internal Resistance of Voltmeter.

Wheat Stone Bridge Method

  • The Wheatstone bridge is a circuit used to compare an unknown resistance with a known resistance. 
  • The bridge is commonly used in control circuits.  For instance, a temperature sensor in an oven often consists of a resistor with a resistance that increases with temperature.
  • This temperature-dependent resistor is compared with a control resistor (outside the oven) to control a heater and maintain a set temperature.

Since VAB = 0, the voltage drop from C to A must equal the voltage drop from C to B, VCA = VCB.  Likewise, we must have VAD = VBD.  So we can write,

Thus, the unknown resistance Rx can be computed from the known resistance Rk and the known ratio.Notice that the computed Rx does not depend on the voltage Vo; hence, Vo does not have to be very stable or well-known.Another advantage of the Wheatstone bridge is that, because it uses a null measurement, (VAB = 0), the galvanometer does not have to be calibrated.

Temperature dependence of resistivity

For this part, the unknown Rx is a copper wire coilAs a measure of how sensitive the resistivity is to temperature changes, we can compute the fractional change in the resistivity divided by the change in temperature

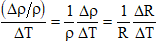Ohmmeter Method

  • The ohmmeter is an electronic instrument which is widely used to check a complete circuit or to measure the resistance of a circuit element.
  • Micro-Ohmmeter, Mega Ohmmeter, and Milli- Ohmmeters are used to measure resistance in different applications of electrical testing.

Series Ohm-Meter

In a series type Ohmmeter, the R1 is the current limiting resistor, the Rx is the unknown resistor, the R2 is the zero adjust resistor, the Rm is the internal resistance, E is the internal battery voltage, and A and B are the output terminals of the Ohmmeter.

In a series type Ohmmeter, the R1 is the current limiting resistor, the Rx is the unknown resistor, the R2 is the zero adjust resistor, the Rm is the internal resistance, E is the internal battery voltage, and A and B are the output terminals of the Ohmmeter.

Shunt Type Ohmmeter

Shunt type Ohmmeter is used to measure small values of resistance.In this type of Ohmmeter, the movement mechanism is connected parallel to the unknown resistance Rx.When the A and B terminals are closed, then the unknown resistor Rx is short-circuited, the needle reads zero because full current flows through the resistor Rx, and I=0 through the meter. Therefore, zero current reading is marked as 0 Ohms.

When the A and B terminals are opened, then the unknown resistor Rx is open circuited, no current flows through the Rx and full-scale current flows through the meter as the resistor R1 is adjusted. So, maximum current reading is marked ∞ Ohms.

Methods For Measurement of High Resistances

  • Direct Deflection methods
  • Loss of Charge methods
  • Megha ohm bridge methods

Megohm Bridge Method

  • We have seen many methods to find low and medium resistances here we discuss methods for high resistance measurement.So now I will explain Megohm bridge method.
  • In the given figure below it shows a very high resistance R with its two main terminals A and B, and a guard terminal, which is put on the insulation.
  • This high resistance R is between main terminals A and B and the leakage resistances RAG and RBG between the main terminals A and B of from a "Three-terminal resistance".

  • The operation of a Mega ohm bridge can be explianed as it is a self-contained Mega ohm bridge which includes power supplies, bridge a member, amplifiers, and indicating instrument.
  • It has a range from 0.1 Mฮฉ to 10⁶ Mฮฉ. The accuracy is within 3% for the lower part of the range to possible 10% above 10,000 Mฮฉ.

Loss of Charge Method

 

  • In the circuit C is a capacitor of known capacitance, V is electrostatic volt-meter, R1 is the total leakage resistance of the capacitor and volt-meter and R is the resistance to be measured.
  • In this method, the capacitor is first charged by means of a battery to some suitable voltage by putting switch S on stud 1 and then allowed to discharge through the resistances R and R1 by throwing switch S to stud 2. 
  • The time taken t for the potential difference to fall from V1 to V2 during discharge is observed by a stopwatch. The equivalent resistance of R1 and R is given as

R’ = t/(C loge V1/V2 ) 

  • From the above expression the value of R’ can be determined. The test is then repeated with unknown resistance R disconnected, the capacitor being discharged through R1 can also be determined.  Knowing the value of R’ and R1 the value of unknown resistance can be determined from the relation

1/R  = 1/R-1/R1

  • While measuring insulation resistance of a cable or a capacitor the test need not be repeated.  In this case, C will be the capacitance of the cable (or capacitor) under test, which must be known (may be determined by any method if not known) and R1 will be the insulation resistance to be measured.  NO external resistance is to be inserted in the circuit.  The value of R1 then can be obtained directly from the expression.

R = t/(C log V2/V1 

This method of measurement is associated with serious difficulties and error such as

  • Leakage and absorption effects
  • Effect of time electrification and
  • Effect of temperature upon insulation resistance.

Direct Deflection Method

 

  • The figure shows the measurement of high resistance using direct deflection method.
  • For measurement of high resistance such as insulation resistance of cables, a sensitive galvanometer of d’Arsonval type is used in place of the microammeter.
  • In fact, many sensitive type of galvanometers can detect currents from 0.1-1nA.therefore, with an applied voltage of 1kV, resistance as high as 1012 to 10*1012O can be measured.
  • The first figure shows the direct deflection method for measurement of high resistance having the metallic sheath.
  • The galvanometer G shows the current between the conductor and the metallic sheath.
  • The leakage current is carried by the guard wire wound on the insulation and therefore does not flow through the galvanometer.


Labels:

Comments

Post a Comment

Thanks for reading my Blogs or articles

Popular posts from this blog

Imagians๐Ÿ“ธ

                                  Marvel's Comics Universe                         Captain Marvel (Movie, 2019) | Official ...                                      marvel.com                                 Marvel Movies ...                                   marvel.com 23 Best Marvel Movies Ranked (2008 – 2019) studiobinder.com Marvel's Phase 1, 2, 3 and 4 explained digitalspy.com Marvel Phase 4: Full list of MCU films ... indy100.com Marvel Movies ... marvel.com How to watch the Marvel Movies in order ... tomsguide.com Marvel United by CMON — Kickstarter kickstarter.co...
Post a Comment
Read more

Bringing Back the Beast: Colossal Biosciences’ Dire Wolf Experiment Sparks Global Buzz

Bringing Back the Beast: Colossal Biosciences’ Dire Wolf Experiment Sparks Global Buzz A futuristic lab with scientists working on DNA editing Caption: Inside Colossal Biosciences’ lab – where science meets the Ice Age In a move straight out of science fiction, Colossal Biosciences, a Dallas-based biotech company, has taken the internet by storm after unveiling three genetically engineered canines designed to resemble the extinct dire wolf. Named Romulus, Remus, and Khaleesi, these pups are part of the company's bold venture into the world of de-extinction.  Side-by-side comparison of a dire wolf skeleton and a modern gray wolf Caption: How dire wolves differ from today’s gray wolves Using ancient DNA from dire wolves that vanished around 12,000 years ago, Colossal’s scientists employed CRISPR gene-editing technology to alter the DNA of gray wolves. They focused on 20 specific genetic sites, adding traits like a larger skull, stronger build, and distinc...
Post a Comment
Read more

THE FIRST COMMUNICATION BETWEEN TWO HUMANS IN DREAMS HAS BEEN ACHIEVED

Pioneering Communication in Lucid Dreams: REMspace's Historic Achievement Silicon Valley startup REMspace has reached a remarkable milestone by enabling the first-ever communication between two individuals in separate lucid dreams. This innovative feat not only expands our understanding of sleep but also opens new avenues for dream-based interaction. How It Works: The Science of Dream Communication On September 24, 2024, two participants engaged in a groundbreaking experiment while they slept in their own homes. Utilizing advanced neurotechnology, their brain activity was monitored as they entered lucid dream states—moments when they are aware they are dreaming and can often exert some control over their dreams. In the experiment, once the first participant became lucid, they received a “Remmyo” word through earbuds. The participant then repeated this word in their dream, and the response was recorded on a server. Shortly after, the second participant, also ...
Post a Comment
Read more

Tumbbad: A Cinematic Journey into Greed, Generations, and Unspoken Political Commentary

Tumbbad: A Cinematic Journey into Greed, Generations, and Unspoken Political Commentary Tumbbad, a haunting and visually arresting film, offers much more than just a thrilling story. While the film has earned widespread recognition for its gripping narrative and unique cinematography, there are deeper layers within the movie that deserve closer inspection. In this blog, I’ll explore how Tumbbad tackles themes of greed, generational legacies, and political commentary that often go unnoticed. These layers elevate it from a mere horror-fantasy to a profound commentary on society and human nature. The Core Theme of Greed At its heart, Tumbbad revolves around one key theme: greed . The entire plot unfolds around this destructive force, shaping characters and their decisions. Vinayak, the protagonist, is driven by an insatiable desire for wealth. His greed for gold mirrors that of Hastar, the ancient deity cursed for coveting both food and treasure. This generational curse of g...
Post a Comment
Read more

Let's Pay Back To Society

A man wearing a torn dhoti and torn shirt arrived at a large hotel with his 15-16 year old daughter. Seeing both of them sitting on a chair, a waiter placed two glasses of clear cold water in front of them and asked - what to bring for you? The man said- "I promised my daughter that if you come first in class 10 in the district , I will feed you a meal in the largest hotel in the city." It fulfilled the promise. Page bring a plate for this. The waiter asked- "What to bring for you?" "He said-" I have only one plate of money. "Hearing the whole thing, the waiter went to the owner and told the whole story and said-" I want to feed both of them a meal. Right now I have no money, so you deduct the bill amount from my salary. "The owner said-" Today we will party the success of this promising daughter on behalf of the hotel. " The hoteliers celebrated a table well with the wedding and very brilliantl...
1 comment
Read more

First Bionic Eye Created by MVG

First Bionic Eye About It Hey guys this is very good news for every one who wants to see the world by there own eyes.And it is done the MVG(Monash Vision Group) of Monash University. They work hard on this project from decades. And they finally succeeded to achieve there goals. In previous decades many scientists found bionic solutions to help people.But it not useful for large scale. And in future we replace the natural eye to bionic eye. And it is fully work on the  Al based system, we see all types of color which human eye can't see . Where it developed Australian University Develops World's First Bionic Eye To fully cure the blindness of any people in the world.It is developed by professors and there team in Monash University. How it Work Basically it transmitted the optic nerves signals to the brain which is very useful area for vision .And brain the signals from Ratina. In this device user should wear a headgea...
4 comments
Read more

Lockdown millionaire Naren Saraf

Naren Saraf success story: opened your own restaurant in lockdown, today earning millions On one hand, due to the Corona epidemic, there was a lockdown in the whole world, many people had lost their jobs, businesses were ruined, on the other hand there were few people who made their success by turning this challenging time into opportunity A new success story has been written. Naren Saraf is one of these people. Those who did not give up even after leaving the job. Today they are earning millions by starting their own restaurant. But it was not so easy for Naren Saraf to do all this. Let's know his story. Naren Saraf hails from Jammu. 23-year-old Naren had dreamed of completing a hotel management course and working in a good place. He wanted to work in the Taj Hotel. During his studies in hotel management, he went to Umaid Bhawan in Jodhpur for an internship. His work there was well liked. He was also selected, but then the world was locked in...
Post a Comment
Read more

Moving Iron dynamometer type wattmeter(electrical measurement part03)

In this article, you will find the study notes on  Measurement & Electrical Quantities-2  which will cover the topics such as  Moving Iron Instrument Working Operation, Radial Vane type & Co-Axial Vane type, Electro-Dynamometer Type Wattmeter, Construction of Electrodynamometer Wattmeter, Rectifier Type Instrument, Factors affecting the Performance of Rectifier type instruments, Advantages of Rectifier Type Instruments & Thermocouple Instrument. Moving Iron Instrument Working Operation The moving iron instruments are classified as: Moving iron attraction type instruments Moving iron repulsion type instruments Attraction Type Instrument Working Operation Moving Iron Instrument Working Principle:  The basic working principle of these instruments is very simple that a soft iron piece if brought near magnet gets attracted by the magnet.  The construction of the  attraction type moving iro...
Post a Comment
Read more

One Man Crore Trees

This person, known as Peepal Baba, has planted more than 2 crore trees to overcome the lack of oxygen, today people have become aura. In today's time, the whole world has been ravaged by the Corona epidemic . Many people have lost their loved ones due to lack of oxygen. There are more clouds of despair all around. In such a situation, there is also a person who has been doing a great job of planting trees on the earth not only at this time but also for the last 20 years. In order to fulfill the oxygen shortage which the world is struggling with and to keep the earth clean, they are planting trees, following social distancing. Swami Prem Parivartan, 48, who lives in Mayur Vihar Phase-1, Delhi, is today known as Peepal Baba. Expressing his love for nature at the age of just 10, he did the planting of saplings. At the same time, he laid the foundation of a larger purpose. B orn in an army in 1966 in Chandigarh , Dr Parvat's first s...
Post a Comment
Read more

11-Year-Old Prodigy Yan Hongsen Shocks the World with 600 Lines of Code for Rocket Building

  In a stunning display of intellect and creativity, 11-year-old Yan Hongsen from China has captivated the world with his remarkable achievement in rocket science. Known as the ‘Rocket Boy’ on social media, Yan has garnered international acclaim for writing 600 lines of code to build a functioning rocket. A Passion Ignited Yan Hongsen’s fascination with rockets began at just four years old, during the launch of the Long March-2 rocket. This early encounter sparked a lifelong passion for space exploration. Currently a Primary Five student, Yan’s early exposure to rockets and astronomy led him to delve deeply into programming, physics, and chemistry. The Rocket Project Yan’s journey into rocket building was not merely a whimsical endeavor but a disciplined project that involved extensive learning and experimentation. Through Chinese social media platform Douyin, where he has around 440,000 followers, Yan documented his progress and shared insights into his rocket-building ...
Post a Comment
Read more